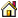Since a few other people have discovered the scintillating mass of colors (I even beat Doom to the punch, before I deleted the post <
×
1: Each of the squares has a slightly darker single-pixel-border around it.
2: The upper left square has an extra border around it.
The RGB values for the squares are also exceedingly interesting, as you can see here:
The top four go as follows:
|Border: |Border: |Border: |Border: |
|78,78,78 |65,65,65 |52,52,52 |39,39,39 |
|Inside: |Inside: |Inside: |Inside: |
|216,216,216|180,180,180|144,144,144|108,108,108|
By itself, this isn't interesting. They're all greys.
Perfect greys, but greys nonetheless. It's normal in
an RGB setup for greys to be perfect numbers. Now
let's add the second row.
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|78,78,78 |65,65,65 |52,52,52 |39,39,39 |
|Inside: |Inside: |Inside: |Inside: |
|216,216,216|180,180,180|144,144,144|108,108,108|
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|78,52,52 |52,78,52 |52,52,78 |78,78,52 |
|Inside: |Inside: |Inside: |Inside: |
|216,144,144|144,216,144|144,144,216|216,216,144|
-------------------------------------------------
Admittedly, it doesn't all break down nicely into rows
--we'll see this pattern fully play itself out in row three. Watch.
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|78,78,78 |65,65,65 |52,52,52 |39,39,39 |
|Inside: |Inside: |Inside: |Inside: |
|216,216,216|180,180,180|144,144,144|108,108,108|
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|78,52,52 |52,78,52 |52,52,78 |78,78,52 |
|Inside: |Inside: |Inside: |Inside: |
|216,144,144|144,216,144|144,144,216|216,216,144|
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|52,78,78 |78,52,78 |78,59,33 |78,33,59 |
|Inside: |Inside: |Inside: |Inside: |
|144,216,216|216,144,216|216,165,93 |216,93,165 |
-------------------------------------------------
Yes, that's right, we've used the same set of numbers
six times, in as many variations as possible. --The
last two are part of the third set, which will make
up the last of the third and fourth row, as seen here:
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|78,78,78 |65,65,65 |52,52,52 |39,39,39 |
|Inside: |Inside: |Inside: |Inside: |
|216,216,216|180,180,180|144,144,144|108,108,108|
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|78,52,52 |52,78,52 |52,52,78 |78,78,52 |
|Inside: |Inside: |Inside: |Inside: |
|216,144,144|144,216,144|144,144,216|216,216,144|
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|52,78,78 |78,52,78 |78,59,33 |78,33,59 |
|Inside: |Inside: |Inside: |Inside: |
|144,216,216|216,144,216|216,165,93 |216,93,165 |
-------------------------------------------------
|Border: |Border: |Border: |Border: |
|59,78,33 |33,78,59 |59,33,78 |33,59,78 |
|Inside: |Inside: |Inside: |Inside: |
|165,216,93 |93,216,165 |165,93,216 |93,165,216 |
-------------------------------------------------
Obviously, because 3P(3) is, in fact, higher than
6, you can't use all the combinations like we could
with the first set, but there you are. I'm not entirely
sure how the numbers were chosen, that's your people's
jobs, I can't even touch notpr0n.
It's interesting to note the first set (The grey set)
uses one number for each square, then the second set
uses two numbers, and the third set uses three.
I don't have the slightest idea what this might mean,
if anything, but it's all I got, and hey, if anyone's
equipped to figure out the riddle of the bar, it's
those guys with 6 goodies.So get to it, I don't want
this to be another Riddle of the Trinkets.
)
, and I have clicked over 400 times. I wonder how many clicks it took those that have goodies. 
Disclaimer: I'm Welsh, left-handed, and stupid. 
