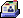×
Take the first player's string and drop the last value. Then take the first value, reverse it, and put it at the front.

Reasoning being this:

In general, either side has a 50% chance of winning.

However, of all winning strings for player 1, only 12.5% will succeed immediately (after three flips). The other 87.5% will be all be preceded by either an H or T. Half of those will be H, half T. In other words, about 43.75% of winning player 1 strings will first contain a winning player 2 combination.

This puts player 2's odds of victory at about 72%.

×If the first person chooses HTH, by that strategy the second chooses THT. These are symmetrical, so each obviously has a 50% chance of winning.

×Case 1: Player 1 picks HHH

Pick THH and you win unless HHH comes up on the first three tosses (chance 7/8)

Case 2: Player 1 picks HHT

Pick THH and you win unless the first two tosses are heads (chance 3/4)

Case 3: Player 1 picks HTH

Pick HHT and you win if the sequence of tosses starts with HH (1/4), THH (1/8), TTHH (1/16) etc (total 1/2) or HTTHH (1/32) and various other possibilities, so the total is over 1/2

Case 4: Player 1 picks THH

Pick TTH and you win if the sequence of tosses starts with TT (1/4), HTT (1/8), HHTT (1/16) etc (total 1/2) or THTT (1/16) and various other possibilities, so the total is over 1/2

The other four choices for player 1 are equivalent to these but with T and H interchanged, so you follow the same strategy but exchange T and H in your choice.

×
The choice to reverse is purely arbitrary -- a strategy that doesn't reverse is just as effective, except that you need to special case it so that you don't end up picking a duplicate.

So another special case to avoid giving Player 1 the first two of your picks in their last two, and you're all set.

×There are eight states (HHH, HHT, ... TTT), each links to two next ones and has two previous ones that link into it. Pick one of the opponent's previous ones thus blocking this path. Don't pick one of his previous ones that he himself blocks with his current choice.

×The first three points must define a triangle, since they cannot be collinear. If the fourth point forms a quadrilateral, then the requirement is fulfilled. If it does not, then the points must form the shape in the diagram (a triangle with a point inside it, with rays drawn through each pair of two points that includes the centre point, extending to the side of the triangle, and indefinetely past the opposite vertex), as that is the only way an angle in the quadrilateral can be greater than 180:



Now, the fifth point cannot lie on any of the lines, for then three points would be collinear. If it lies in one of the external regions, then it will form a convex quadrilateral with the two points bordering the region, and the centre point, because the only way for any angles to reach or exceed 180 degrees would be to either lie on a line, or lie in a different region, in which case this step should be repeated.
If it lies in one of the internal regions, then draw two lines to the points bordering the region. The angle formed must be less than 180, else it would be on a line, or be in a different region. This point is now external to one of the three triangles formed by the original four points - namely, the external triangle that includes the two points bordering the region. Thus, it forms a quadrilateral with this triangle. The angles are restricted to less than 180 degrees by the region boundaries, unless the triangle was completely flat, in which case all points would be collinear anyway. Therefore, it forms a convex quadrilateral, and the requirements are met.

×One hundred and ninety-eight thousand, eight hundred and eighty eight; one hundred and ninety-eight thousand, eight hundred and eighty-nine; one hundred and ninety-eight thousand, eight hundred and ninety; and one hundred and ninety-eight thousand, eight hundred and ninety-one work, if I'm not mistaken. (That is, 198,888, 198,889, 198,890, 198,891.)

×
199999
200000
200001
200002

×a,e,i,o,u,y.

×Arbeitsforum
Humoristenpack

×absentious
un-occidental

... but both of these are cheating a little: the first has a digraph and thus contains only four vowels*, and the second is a derivation that looks like it ought to be valid, but still seems unlikely in the wild.

Ah, update. If you want your own chance at solving this puzzle, do not do the google corpus thing for "unoccidental" -- turns out it occurs exclusively in answers to this puzzle, and most pages containing the word will also have a list of other solutions that you probably don't want to see.

* Edit. I see now that the reduced sample word given in the puzzle was "enough", which has the same problem. So, half solved I guess.

×uncomplimentary

×"Facetious" (or facetiously) for the a-e-i-o-u-(y) order; and "subcontinental" for the reverse order.

×I think, therefore I am.